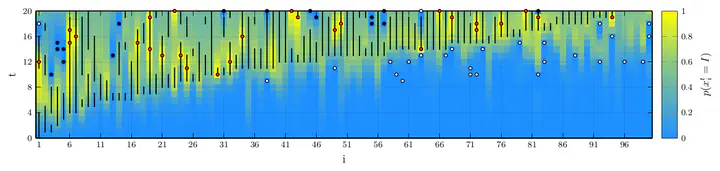
 Inference of an epidemic generated by a SIRS model.
Inference of an epidemic generated by a SIRS model.
We developed a novel generalized mean field approximation, called the Small-Coupling Dynamic Cavity (SCDC) method, for Bayesian epidemic inference and risk assessment. The method is developed within a fully Bayesian framework and accounts for non-causal effects generated by the presence of observations. It is based on a graphical model representation of the epidemic stochastic process and utilizes dynamic cavity equations to derive a set of self-consistent equations for probability marginals defined on the edges of the contact graph. By performing a small-coupling expansion, a pair of time-dependent cavity messages is obtained, which capture the probability of individual infection and the conditioning power of observations. In its efficient formulation, the SCDC algorithm is linear in the duration of the epidemic dynamics and in the number of contacts. The SCDC method is derived for the Susceptible-Infected (SI) model and straightforwardly applicable to other Markovian epidemic processes, including recurrent ones. It exhibits high accuracy in assessing individual risk comparable with Belief Propagation techniques, outperforming heuristic methods based on individual-based mean-field approximations.